Scientists have always looked for theories and proofs that are pleasing and satisfying. It is a natural desire that stems from human aspirations to perfection. For example, according to calculus, adding 1+1/2+1/4+1/8…¥ = 2. This seems intuitively correct, and, as a consequence, science often sets out to prove such theories. In so doing, small errors are often encountered but ignored, being put down to inaccuracies in scientific method or measurement. For many centuries, mainstream science attempted to carry out experiments that excluded interferences such as friction. Friction and other non-linearities (see appendix 3) were considered a form of imperfection. Friction, however, is to be found everywhere in the world and known universe. The orbit of the moon is affected by friction created by the oceans, and simple everyday actions such as walking would not be possible without it. Eventually, science came to a point where non-linearity could not be ignored.
Logic tells us that adding up the individual parts should give us the whole. But what we also know from experience is that there are critical points where a small movement has a disproportionately large effect. Earthquakes offer a prime example. The slow but steady displacement of the earth’s tectonic plates has been creating a tension between two surfaces. For many years nothing happens, friction prevents any offset movement between the surfaces. Then a critical point is reached. One plate moves an additional fraction of a millimetre and New Zealand experiences a severe earthquake that destroys hundreds of building and kills ten people (and fifty sheep!). Looking on a microscopic scale, it could be said that the tectonic plate moving a fraction of a millimetre caused the earthquake. Although this is true, it does not make sense without also considering the years of prior displacement. Chaos theory helps to explain this.
Unlike other scientific discoveries, where developments are individual achievements, chaos theory was discovered and explored by many scientists from varying backgrounds. Some of the earliest, like Poincaré, Julia, and Fatou, did not have available to them the technology to make their findings renowned, and receded into the background. Since the advent of the computer, however, the calculating power has been available to expose chaos with broad application.
As far back as the late nineteenth century, a physicist, mathematician and philosopher from France called Henri Poincaré saw the possibilities of deterministic chaos existing inside closed Newtonian systems. A pendulum swinging in a vacuum, not impacted by friction in any way, is seen as a closed system, that is, it has no outside influences. In Poincaré’s era, any disorder in a system was seen as an outside influence that would disappear if it were possible to emulate a closed system environment. Poincaré’s work was one of the earliest contradictions to these views.
Newton’s laws of planetary motion are capable of predicting the orbits of two planets in a closed system environment, for example in a universe consisting only of the earth and the moon. Matters become slightly more problematical, however, if a third body is added to the equation, for example, the sun. In fact, according to science writer, John Briggs and physicist, F. David Peat, Newton’s equations become unsolvable.
In order to solve equations involving orbits of more than two bodies, a series of ever-smaller approximations are used to arrive at the answer. Poincaré contemplated what would happen if these approximations had an impact over a long period of time. Looking at the problem mathematically, it was non-linear, but nonetheless, it appeared that the introduction of the third body had little effect, for the most part. He did, however, discover that certain orbits caused a planet to wobble and then fly off course, even out of the solar system. This could have huge implications for our solar system if over time, a series of planets happened to end up in one of Poincaré’s chaotic orbits; planets could suddenly start flying out of the solar system. Poincaré introduced one of the hallmarks of chaotic behaviour in an essay called "Science and Method" in 1903: ‘sensitive dependence on initial conditions.’
At the time, however, Poincaré’s discovery was largely ignored as it was overshadowed by many of the other great scientific discoveries of the early twentieth century. Max Planck’s work on quantum theory was challenging Newton’s theories and Einstein was presenting his theory of relativity. Poincaré himself left his research, feeling overawed by his "bizarre" discovery.
Later, in 1954, three Russian scientists, A. N. Kolmogorov, Vladimir Arnold and J. Moser, collectively known as KAM, provided some of the answers to Poincaré’s problem. Firstly, they noted that the condition Poincaré described could not occur if the third body had a gravitational pull less than that of a fly on the other side of the world. Secondly, they noted that it could only occur if the cycle of the planet’s orbits fell in a ratio, that is, they repeated over a period of time. This means that the effect the third planet would have is one of positive feedback (see appendix 1), and, therefore, the change is amplified over time. If this is not the case, and the planet’s orbits are quasi-periodic, then it demonstrates a form of negative feedback and is self-corrective.
What this positive feedback system shows is ‘deterministic chaos’. This contradicted and nullified the idea that chaotic behaviour could not occur in a closed system.
Poincaré and KAM’s theories are also backed up by evidence in our own solar system. Holes in the asteroid belt have been found where the latter coincides periodically with the orbit of Jupiter. Asteroids once in these zones have been sent flying randomly off into space. Some of the asteroids that have collided with the earth could be accounted for by such a theory.
According to Jack Wisdom of the Massachusetts Institute of Technology, many of the moons in our solar system must have undergone some periods of chaotic behaviour in the past but have since developed quasi-periodic orbits. One of Saturn’s moons, Hyperion, appears to be undergoing one such period at the moment. Gaps in Saturn’s rings are also possible results of KAM theory, although research in this topic is still under way.
4.2 Gaston Julia and Pierre Fatou
Throughout history, developments in geometry have run parallel to advances in other areas of science. The architecture and surveying of the Egyptians was only made possible by advances in geometry. The Greeks made many geometrical developments and applied them to practical science. For example, they were able to determine the distance of a boat from the shore using Pythagoras’ theorem. They were also able to light fires using parabolic mirrors by focusing the sun’s energy. In fact, according to Benoit B. Mandelbrot (the famed ‘inventor’ of fractals), Johannes Kepler’s seventeenth century discovery that orbits of planets could be described as ellipses was a catalyst for Newton’s work on gravity. More recently, fractals have arisen as one of the leading geometrical fields. Crucial to their inception was work by Poincaré and the duo of Gaston Julia (1893 – 1978) and Pierre Fatou (1878 – 1929). They studied the dynamics of complex number maps around 1910, developing Julia Sets and laying the groundwork for modern fractal imagery.
Julia was an Algerian born mathematician. He had the misfortune of loosing his nose in World War I and carried out much of his mathematical research in hospital. Julia worked on iterative functions whereby fn(z) stays bounded as n tends to infinity (where z is a complex number). Much less is known about the life of Fatou, although his work involved planetary motion. As with Julia, he had particular interest in rational functions with complex variables. Without the iterative power of computers, however, their research was limited and after a short period, the study was all but forgotten until the 1970s when Mandelbrot rekindled interest in the subject.
Benoit Mandelbrot was a Polish-born mathematician who, from the outset, had adopted an unconventional view. His educational background was also unconventional in that he claimed never to have learnt the alphabet or multiplication tables. He had a difficult childhood, fleeing from Nazi prosecution, due to his Jewish background.
In 1936, the family moved to Paris, where Benoit’s uncle, Szolem Mandelbrot, lived. Szolem was a mathematician and a founding member of Bourbaki. Bourbaki was a mathematical ‘cult’, designed to rebuild mathematics after World War I. Bourbaki’s attitude was directly opposed to that of mathematicians like Poincaré who said, "I know it must be right, so why should I prove it?" Bourbaki moved away from mathematics as a means of explaining physical phenomena, believing that mathematics was a science of its own and that it should not be judged by its application to other sciences. Indeed,
One of Mandelbrot’s ideas that gained him most notoriety was the paper, "How long is the coast of Great Britain?" – a seemingly trivial question that yields the surprising answer – ‘infinite’. When measuring the length of a coastline, the results depend on the detail of the measuring. If measuring a coastline from a satellite photo, one might choose to pick a point every hundred metres around the coastline and measure between these points. However, if greater accuracy is desired, a point every fifty metres may be measured. Although the coastline is still the same, the distance measured now proves to be greater, as more of the bays have been taken into account. The more detail in which we look at the coastline, the longer the coast becomes. Hence, at an infinite level of detail, the coast is infinitely long.
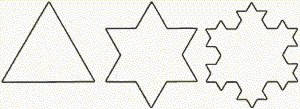
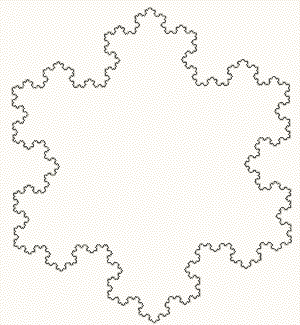
A simple geometric representation of this idea can be seen in the Koch Curve, described in Mandelbrot’s words as "a rough but vigorous model of a coastline." The Koch curve is made up of equilateral triangles. An equilateral triangle one third of the size of its originator stems off each triangle. Therefore, the length of the perimeter of the Koch curve is 3 * 4/3 * 4/3 * 4/3…and ad infinitum.


Looking at the Koch curve from a distance, it appears that it is merely a twelve-sided star, or two superimposed equilateral triangles. Each ‘edge’ is as follows: moving closer in, the overall shape is maintained but the detail (and length of ‘coastline’) increases. If a section of it is magnified sufficiently, it appears identical to the original image.
This idea of self-similarity on differing scales is an idea central to fractals (see section 5.2.1).
Ever since the early days of modern computers, meteorologists have seen them as an extremely useful tool. One such meteorologist was Edward Lorenz. He was born in America in 1917 and worked at the Massachusetts Institute of Technology. He had set up a computer in his office that could simulate hypothetical weather for 12:00 am each day. It was a very simple model that worked on twelve variables, such as air pressure and wind speed. Each minute, a day would pass and the computer would produce a print-out telling Lorenz the weather. Despite its simplicity, it was a surprisingly realistic simulation, showing various patterns, although with a certain irregularity.
In 1961, Lorenz made the accidental discovery that small disturbances in initial conditions could result in unrecognisable results (see section 5.3). Through his chance finding, he was one of the first to stumble across deterministic chaos and fully realise its ramifications.
He termed this ‘the butterfly effect’; the small disturbance caused by a butterfly flapping its wings could be enough to create a storm on the other side of the world a few days later.
Robert May was born in Sydney, Australia, and started his career as a physicist, before studying applied mathematics at Harvard. Following this, he developed an interest in biology at Princeton University in New Jersey. Because of his mathematical background, he brought a new approach to the study of population modelling, resulting in his investigation of bifurcations (see section 5.1.1). Using mathematics to model populations, May examined an equation as a whole, rather than looking at individual values. Previously, scientists had been trying to comprehend individual values in an attempt to find patterns and predictability. May was moving away from reductionism towards a holistic approach.